Place Value: An Essential Understanding

No, this ain't your momma's math... It's better!

Mathematics education has come a long way over the past 10 years. The calculations, of course, are the same (after all, 33 + 20 still equals 53), but teaching mathematics has certainly evolved. 

"Back in the day" knowing that the answer was 53 was typically all that teachers expected – it was mostly about getting the correct answer – I honestly cannot remember a single time that a math teacher asked WHY my answer was what it was. Of course, the correct answer is still important, but mathematics education of today requires that learners understand why the answer is 53 – we want our students to deeply understand the patterns and relationships of numbers that allow for greater flexibility and application of the mathematics. In other words, we are teaching our students how to become thinkers of mathematics, not just do-ers of math. 

Let's focus on PLACE VALUE. Did you know that "place value" is mentioned 25 times in the Common Core Standards in grades K through 5? Take a look at a brief progression of place value through the grades:  

That's good information about the progression of Place Value learning, but WHAT DOES IT LOOK LIKE in the classroom?

Before students even begin adding and subtracting multi-digit numbers in 2nd grade, they should first learn how to be flexible in the way they decompose numbers. Take the number 53, for example. In many 1st grade classrooms, students learn to break 53 into 50 + 3... BUT they also need to understand that 53 can be represented by 53 ones, or 4 ten and 13 ones, or 40 + 13 ➥ this flexible way of thinking about 53 lays the foundation for the work they will do in 2nd grade. 

Imagine students are posed with the problem 53 – 27.

What if instead of just recognizing 53 as 5 tens and 3 ones (which poses a problem when they try to imagine taking away 7 ones), they also could easily imagine 53 as 4 tens and 13 ones: 

Being flexible with place value gets even more exciting when we subtract larger numbers – this is how those who are mathematically fluent have been solving problems like this for generations (in spite of what they were being taught in school) – it's us educators who are finally catching up to them!

We also saw in the progressions that rounding number relies on an understanding of place value, too. Think about the number 1,742.  By understanding place value, we understand that

• 1742 has 1742 ones
• 1742 has 174 tens -- yes, I know there's a 4 as the tens DIGIT, but there are not just 4 tens - there are 174 tens (and 2 ones). 
• 1742 can also be thought of as 17 hundreds (and 42 ones) – Let's read that one again so we can process it fully – 1742 has 17 hundreds (not just 7 hundreds). 

The ability to think flexibly about place value in this way makes rounding much easier to understand. Imagine we were asked to round 1,742 to the nearest 10.  By knowing this number has 174 tens (and a few extras), we understand that this number has a little more than 174 tens (because of the few extras) but less than 175 tens. Of all the numbers in the world, we have narrowed it down to just two specific numbers that this number must round to – either 174 tens (1740) or 175 tens (1750).  Then by looking at the "extras", we know that we don't have enough to make it halfway between those two numbers (to 1745), so we must round to the lower value 1740 when rounding 1742 to the nearest 10. 

Place value understanding is foundational to building a deep understanding of numbers and supporting fluency. We didn't delve into it this time, but place value should be explored and learned using concrete models. Here are a few images from other educators for inspiration as you continue on the place value journey with your students!

The NUMBER LINE – A Hidden Treasure in Plain Sight!

Just about every elementary math classroom has one - I've worked in three different school districts in my career and having a number line displayed on the wall has been the standard in each of those districts. Let's pause for a minute and think about that phrase "displayed on the wall"....

WALL  ART

or

LEARNING  CHART

A number line display? Is that really what we want? A display rather than a tool. I want my number line to be a tool – a number line is a powerful learning aid when it's used. Now think about where most teachers hang their number lines... in just about every classroom, the number line is hung on the wall wa-a-y-y up high in a location where access is challenging to our young learners who benefit from using the number line as a hands-on learning tool. 

Why do we hang this amazing math tool in a place where access is difficult? Lack of wall space is certainly one of the reasons, perhaps it's just become a classroom tradition, and maybe we just don't understand the full value and the range of applications that support instruction when using a number line. 

Many teachers have already discovered one of the solutions: Keep your wall art for reference BUT make sure students have hands-on access to other number lines (perhaps one is taped to their desk or maybe various number lines have been placed in their hands-on math kits). Okay, so now that we've brought the number line down to students, let's talk about a few of the many amazing (and perhaps surprising!) ways to use a number line to support learning. 

AMAZING (and perhaps surprising!)
WAYS to USE a NUMBER LINE to SUPPORT LEARNING

 

Did you know that the CCSS Standards which have been adopted by many states, specifically names "Number Line" as as strategy/model 30 times (!). In addition to those 30 callouts, the number line can be an effective modeling tool for many other learning moments, as well. 

Many classrooms already use the number line to model addition and subtraction, one more and one less, counting and before and after concepts, but what about multiplication? division? elapsed time? unit conversions? and rounding? Can a number line be used effectively to solve for these types of problems? 

Yes, yes, yes, yes, and yes! 

MULTIPLICATION

If we know 4 x 20, how could we use the number line as a tool to visualize 4 x 19? Those 4 jumps of 20 would be 4 jumps of 19, so we'd need to compensate for the 4 big jumps by backing up 4 (1 for each 20 that was really a 19). What if we were calculating 4 x 18? How could we use our visual model of 4 x 20 to solve that equation? Are you thinking we could do 4 x 20 then back up 8 (compensating for the 2 extra spaces with each jump of 20)? What if the original problem had been 5 x 19 instead of 4 x 19? How might the number line model be the same? How might it be different? 

DIVISION

I love using the number line model for division. You'll likely notice that this is just a partial quotient method that utilizes the number line. Many students (and teachers!) find this number line model easier to understand and manage with division equations than using the box method or area model. 

Notice how we take advantage of multiplication facts that we know to get to the quotient. Take a look for yourself, what do you think? 

ELAPSED TIME

A number line is a simple way to straighten out our traditional analog clock.  We can use a number line model by creating friendly jumps to make calculating elapsed time more efficient. 

Check out the two models below that help students (adults, too!) calculate elapsed time. Because I use the number line model so much, I can now visualize a number line in my head when trying to figure out what time I will arrive at my destination 🚘.

How are these two models the same? How are they different?

Now we simply add the values of the  friendly jumps to calculate that the workout was 38 minutes long (that's not a bad workout on a busy day, right?)

 

ROUNDING

This is a procedural TRICK!
This is NOT a good model

I Googled rounding strategies the other day and found mostly a bunch of rounding tricks (mnemonic devices to remember and various rhymes - some that were so complex that I couldn't see how that possibly made it easier!). Anyway, we don't need tricks – tricks are just that, ways of tricking people into believing that they know or understand a concept. We need to teach strategies that actually help students to conceptually understand the mathematical concept; after all, we want them to understand the relationship of numbers and then generalize their understandings in other applications, right?

The number line model can help us give relational context to a number. Imagine we wanted to round the population of Palm Springs to the nearest thousand. I can see that there are 44 thousand (plus a few more) people who currently reside in Palm Springs. The question is whether the "plus some more" puts us closer to the next thousand???

The Critical Importance of Benchmark Numbers 

Benchmark numbers are the KEY to UNDERSTANDING rounding numbers. 

In the model below, I know 44 thousand (plus) live in Palm Springs. So the value is more than 44 thousand but less than the next higher thousand (45 thousand). These values become my bookends. The benchmark value that falls exactly halfway between the two values is 44,500. 

Next I consider if my number is approaching 44,500 (making it closer in value to 44,000 rather than 45,000), or has my value of 44,612 already passed my halfway benchmark of 44,500 and is now closer to 45,000?

Think about how this method builds a conceptual understanding rather than relying on procedural memory, or worse, a catchy rhyme that requires no understanding of the math that drives the math. 

CONVERSIONS

I love using a double number line for conversions. I first started using this model when I learned that 24K jewelry is made of 100% gold, and being the mathcurious person I am, I wanted to figure out the percentage of gold in my 14K gold wedding ring. 

Recently, a group of learners used the number line model for a conversion related to a Washington DC landmark: The Washington Monument. 

Let's begin with a bit of background for the problem: The Washington Monument was built in two phases. The first phase was 152 feet tall, but they ran out of money to complete it, so the monument stayed incomplete for two decades until Congress decided to pass a joint resolution in 1876 to complete the project. The completed monument is 555 feet tall - at the time, it was the tallest structure in the world. 

Back to the math.... As you study the progression of the model, you will notice they reflect the progression of thinking. 

How does this number line model for unit conversions scaffold thinking better than the algorithm method of solving the problem? 

How can we use 1 foot = 12 inches to determine how many inches are in 100 feet?

 

How does knowing 100 feet, help us quickly know the number of inches in 50 feet? 

We have the conversion for 100 and for 50, that's 150 of the 152 feet. 

We just need 2 more feet.

How did we determine that 152 x 12 = 1824 without actually multiplying 152 and 12?

What math concepts can you teach using the Number Line Model to build students' conceptual understanding of the relationship that numbers share?

Effective Questioning in the Classroom

PUSH or PAUSE

I recently had the pleasure of joining district supervisors and building administrators on some instructional walk-throughs in each of the elementary schools in my district – it was wonderful to see all the good instruction and relationship-building happening in the learning environments across the district. In one particular classroom, I was acutely aware of just how skilled the teacher was at facilitating student thinking by making in-the-moment-decisions about when to PUSH and when to PAUSE. 

Teaching is often (and accurately) described as both a science and an art – and when the two work together seamlessly, well, that is when the magic happens! But it's not really magic, is it? It's a skilled teacher who understands the important balance between effective Questioning and effective Wait Time. It doesn't matter if the teacher has 3 years or 30 years behind them – this is something we can all learn to do when we work to be purposeful about our craft. As I spent time in this classroom, I couldn't help but notice how this teacher had found that perfect balance. I found myself trying to figure out how to bottle it and share it with others, knowing then that it would be the topic of my next post – this post.

So what does it mean to strike a balance between PUSH and PAUSE? And what does this balance look like in the classroom? We should probably begin by defining the terms PUSH and PAUSE, so we have a shared understanding of what those words mean as it relates to this post. 

PUSH is when you question students and push them beyond their initial answers. Through effective questioning, you encourage them to analyze their own thinking and then push them to say more, explain more clearly, provide more detail, and articulate their thinking clearly for others to understand those thoughts they have swimming around in their heads.  A previous MathSnack post highlights specific push moves to encourage deeper student-thinking. 

PAUSE happens when you simply give students a bit more time and space to analyze their own thinking and find the ideas and words to articulate that thinking. They don't need another question – they don't need a push – they just need time to process the ideas already firing the synapses in their brains and trying to find their way out into the room. 

Though I can't do justice to the actual lesson, perhaps a short snippet from the lesson will help explain PUSH and PAUSE a bit more clearly:

During this class period, these young 2nd graders were learning about line plots. This was their introduction to seeing data represented on a line plot. The students discussed measurement the week prior and were now learning how to graph measurement data on a line plot. The teacher asked, "Why does this line plot end at 52?" She then provided PAUSE for students to consider her question. She was not expecting an immediate response, nor did she want one. After a few seconds, she prompted students to talk about their ideas with their Turn-n-Talk partner – clearly an established routine in her classroom. Students began talking about why the line plot ended at 52 with their partners; they even went further to discuss why it began at 48 instead of zero.

As students talked to each other, the teacher circulated the room just to listen to conversations and gauge her students' initial understanding of line plots. She waited just long enough for the conversations to generate ideas, but not enough time for the conversations to lag or wane. She then directed students' attention back to the data on the screen, calling on a few students she had noted had meaningful contributions to add to the discussion. The first student she called on replied, "It's the greatest."  The teacher PUSHED and asked the student, "What do you mean by that?" How easy it would have been to just affirm the student's response by saying, "Yes, 52 is the greatest value of length in inches from the data we have." Many teachers would have given the student credit for words he did not actually say, but this teacher PUSHED, required the student to find his own words, and expected him to articulate his own ideas so others would also understand. The student thought briefly and then responded with a deeper, more thoughtful response that enriched the discussion. 

The teacher's flow was natural, comfortable, and produced great results. She asked her students to consider a question (e.g., "What do you notice about the line plot?"). After she posed each question, her students knew she would leave room for PAUSE so they could consider the question and their initial ideas about it. She then asked them to talk through their ideas with their Turn-n-Talk partner, giving everyone in the room an opportunity to develop and share their thoughts rather than just one or two students who would have typically been called upon to answer (see more about the critical importance of Practice Turns and Feedback here). Once students had an opportunity to talk with a peer, she opened the discussion to the whole class and encouraged students to compare their ideas to others being presented in the room. Many students used silent hand signals to indicate that they had a similar idea to the one being shared. When needed, the teacher PUSHED students' responses with questions that challenged them to connect the mathematics and fully articulate their ideas. The conversations were meaningful, the questions promoted deeper thinking, and the students rose to each challenge given to them. It would have been easy to forget that this was a class of 7- and 8-year-olds in a 2nd grade classroom. But instead, I left wondering how I would be able to explain this beautiful balance of PUSH and PAUSE – I am still wondering how I can bottle it up and share the experience with others. 

22 Ideas to Celebrate Super TWOsDay

For my school district, the 100th day of school falls on February 11th this year – perhaps you have or are planning to celebrate the 100th day of school with your students, too... 

FUN FACT: The 100th Day of school is actually the 1000th day of school for 5th graders - check the math: Kindergarten + 1st + 2nd + 3rd + 4th = 5 x 180 days = 900.  Now add the first 100 days of 5th grade (900 + 100) and that equals 1000 days!  

This year, less than two weeks after the 100th day of school, we will have an opportunity to celebrate a day that is TWICE as exciting: Super TWOsDay! 

Super TWOsDay is February 22, 2022 and it falls on a Tuesday! The next time that February 22 falls on a Tuesday will be in 2028, then 2033, 2039, 2050, and 2056 (none of those are nearly as exciting as 2/22/22 since the years are not also 2s).

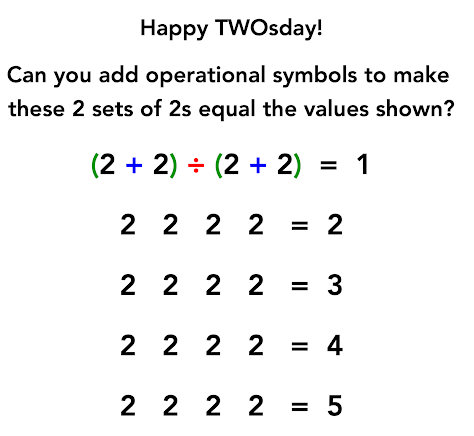
I DOUBLE dog dare you to try out a COUPLE of the ideas on this page with your students – Perhaps, you'll want to set a goal of doing at least TWO Super TWOsDay activities. Keep scrolling to check out these 22 ideas to celebrate Super TWOsDay with your students in ways that are t(w)otally awesome!  (ugh! that last attempt at "two humor" was bad, wasn't it?)

IDEA #1 (for grades 3+)

Here's a fun challenge for your class to try on this very special TWOsday.

DOUBLY DIFFICULT: Try to create values up to 10 using the 2 sets of 2s

I wonder if there is more than one way to make each value?

Making 9 was tricky for me, but I figured out a clever way to do it 😉 

HELP! Now I'm struggling to make a value of 7

I'd 💖 to see your students' solutions to this TWOsday challenge

IDEA #2 (for everyone!)

TWOsDay-INSPIRED WARDROBE: Encourage your students to dress up in "2-themed" outfits: Tutus (get it?), wear 2 bows, dress like a twin with a classmate. I plan to wear my new TWOsday inspired t-shirt. If you want your own TWOsday tee, you can find dozens of super cute designs with simple Google search. My fact-based brain couldn't resist buying a design that offered a list of additional number facts about this day and year - found this one on RedBubble. 

Got my long sleeve TWOSDAY tee today!

IDEA #3 (for grade K-2)

HUNDREDS CHART HUNT for 2s – Give each of your students a hundreds chart (or 120s chart). Challenge students to find and color in all of the numbers that have the number 2 as a digit. THEN discuss any patterns that they notice [the second column is colored in because there is a 2 in the ones place for all of them, 20 and 120 are both highlighted, the 20's row is highlighted except for the last number 30]. Be sure to discuss why these patterns occur as it relates to place value. Then ask them, "If this chart went on, what would be the next number that we would color in?"

IDEA #4 (this one is likely best to use with upper grades)

Same 120 chart as shown above, but for this one, challenge students to use the digits of each number to find a value equal to 2. Here are a COUPLE of examples (yep, I'm going to squeeze every "two"-related reference I can think of into this post!): 

• 13 - using the digits of 13, we can subtract the digits 3 – 1 = 2
• 97 - using the digits of 97, we can multiply 9 x 7 = 63 and then use those digits 6 ÷ 3 = 2

I wonder how many of the numbers could be eliminated in this challenge?? Could we figure out a way to eliminate ALL of them? Or perhaps the challenge is to find a "2" calculation with 22 of them - ahhh, that we could probably do with just a bit of collective effort!

IDEA #5 (can be adapted for any grade)

MEASUREMENT ACTIVITY: Ask students to find items that are exactly 2 units long. The units could be inches, feet, paperclips, shoes, ... be creative! Keep a record of your items. Compare measurements with other students. Allow time for students to discuss their observations. 

ASK: How can all of these things have a measurement of 2 units if they are different sizes? This is a great activity to explore how the size of the unit impacts the relative length. 

Could we create a new unit of measure where 2 of the larger unit equals 22 of the smaller unit? What would that look like?  [Did you just think about how each unit would be partitioned into elevenths -- see how a simple, purposeful question can generate so much mathematical reasoning and discussion?!!]

IDEA #6 (pick and choose the questions that will challenge your students appropriately)

QUICK IDEAS: 22 quick cross-curricular ideas to do in the moment (click HERE for a printable version of these 22 quick tasks)

IDEA #7 

VIDEO FUN: Show a video celebrating the number 2 during your TWOsday celebration

(grades PK-2)

• Doubles! Doubles!
• The Number 2 Song
  
• Jack Hartmann's Dancing 2s
  
• Jack Hartmann's I Can Show the Number 2
  

(grade 3)

• Jack Hartmann's Multiply by 2
  
• Catchy Multiply by 2 Song
• 2 Times Table Song

IDEA #8 (for everyone!)

BREAK TIME: Take several brain breaks that also DOUBLE as exercise breaks. With each break, try to do 22 of the named exercise. Need a few ideas? Try these:

• arm circles (22 forward circles / 22 backward circles)
• hop on one foot (22 hops on each foot)
• jumping jacks
• toe touches
• high knee marching
• run in place for 22 seconds

IDEA #9 (for grades 3+)

A 2-INSPIRED MATH GAME – This game builds number sense: Roll two dice to get the digits for your starting number (for example a 4 and 5 would be 45 or 54). Students write the number and then DECIDE if they should ADD 22 or MULTIPLY by 2 to get the greater value. Keep a chart of the trials. Roll/record/calculate/repeat for at least ten numbers. Challenge your students to discover if there is a pattern of which operation is better to yield the greater value [SPOILER ALERT: If the number is less than 22, it is better to +22. If the number is greater than 22, it is better to x2. If the number is exactly 22, the result is the same with either operation. Once students discover the pattern, be sure to discuss WHY it's true - the reflection discussion is where the power of just about any activity is found!]. 

IDEA #10 (for grades PK-2)

READ ALOUDS: Why not have a TWOsDay Read Aloud event? There are a lot of books that fit the TWOsDay theme – check your library or click on one of the links below for a video real aloud:

• Just Right for Two by Tracey Corderoy and Rosalind Beardshaw  – a story about needing friends – click the title for a video reading of this book
• A Tale of Two Beasts by Fiona Roberton – a fun book about two perspectives
• Double the Ducks (PK-K) by Stuart J. Murphy – a story for PreK and Kindergarten students about the important math skill of doubling
• Two of Everything by Lily Toy Hong - A Chinese folk tale that also teaches a lesson about multiplying by 2s

IDEA #11 (for everyone!)

TWOsDay GIFT: Give each of your students a #2 pencil as a Super TWOsDay gift - look for brands that have the "2" clearly visible but don't waste your money on those horrible pencils that have terrible graphite inside - Ticonderoga pencils really do rule! and I hear the Amazon Basics are pretty good, too. 

Image Source: https://lifehacker.com

IDEA #12 (best for grade 5 - you'll likely have to stretch their knowledge base by explaining squares and roots) 

MATH PUZZLE: Click HERE to get this challenging TWOsDay math puzzle from Mashup Math

IDEA #13 (can be adapted for most grades)

WRITING IDEA: Just for fun, I'll throw in 2 ideas that are not math-related but still make great TWOsDay activities for your class. Perhaps a WRITING idea for this special TWOsDay:

• Write about TWO people who have had a positive influence in your life 
• Complete a pictorial graphic for the theme "When I'm 22". Where will you live? What is your job? What do you like to do? 
• If I had $22 to buy someone a gift, I'd....

IDEA #14

READING IDEA: Here is the SECOND not-so-mathy idea: Challenge your students to read TWO books in the TWO weeks prior to 2/22/22. When Super TWOsDay arrives, invite your students to bring their books for a partner book talk (that's TWO people). Ask each of your students to tell their partner what their book was about, what was their favorite part of the story, etc. 

IDEA #15 (for everyone!)

I SPY 2: Why not play "I Spy" while walking through the halls to lunch, recess, PE, etc.  As students walk through the halls, ask them to look for the number 2. When they see one, they should raise their hand holding up 2 fingers, of course! Pause to notice all the 2s around your building.  Be sure to include conversations about how that two is being used and talk about the place value of each 2 you find - after all, the 2 that  you may find on room 211 is very different than the 2 you'll find on room 112 or on a clock or in a phone number.... 

IDEA #16 (geometry for all!)

2-D DESIGN: Design a picture using 2D shapes: circles, squares, triangles, rectangles, trapezoids.

Idea Source: https://kidsactivitiesblog.com/50959/art-math-inspired-klee/

IDEA #17 (grade 5 standard 5.OA.B.3)

Ask students to (1) generate a pattern that is related to the number two, (2) create ordered pairs using their "2-rule", and then (3) graph the pattern.  Post all of the graphed patterns and discuss how using each 2-rule impacted the graphed lines. Students might, for example, DOUBLE the x-value to get the y-value. Or perhaps the y-value is equal to x + 2. Encourage students to be creative with their 2-rule. The power of this activity will be in comparing the graphs to see how the various 2-rules impacted the graphs. 

IDEA #18 (grade 5 standard 5.MD.A.1)

In grade 5, students are asked to convert units of measure, such as yards to feet and centimeters to meters to centimeters. On this very special TWOsDay, present students with various measurements that are "2-something" (i.e. 2 feet, 2 pounds, 2 miles) and ask students to convert the measurements into equivalent values. Don't think worksheet, think engagement.... Perhaps begin by creating a student-generated list like the one below:

• My house is 2 MILES away from the school
• My cell phone is about 2 INCHES wide
• My guinea pig weighs about 2 POUNDS

Once they have a class list, challenge them to convert the "2" measures into equivalent values either by providing them a reference sheet or encouraging them to look up various measurement conversions (I favor the latter if students have access to do a few in-the-moment searches on the internet). 

• My house is 10,560 feet away from school because 1 mile is 5,280 feet so 2 x 5,280
• My cell phone is about 0.16 feet wide because 1 foot is 12 inches so 2 ÷ 12 (if you're wondering, yes, absolutely permit calculator use – we are digging deeper into unit conversions, not assessing their ability to divide fractional values)
• My guinea pigs weighs about 32 ounces because 1 pound is 16 ounces so 2 x 16

IDEA #19 (grade 4 standard 4.NF.A.2)

Ask students to generate 2 different fractions that each contain the number 2 [i.e. 1/2 and 2/3]. Then ask students to create models of these two fractions [definitely plan to use hands-on fraction models or try out these virtual fraction tools – I love the ones found on Toy Theater and Math Learning Center]. Encourage students to discuss the comparisons. 

 

IDEA #20 (grade 4 standard 4.NBT.B.6)

When students can write their own word problems, this demonstrates a higher level of understanding and thinking. Challenge students to write multiplication/division word problems with one of the values in the problem being 22, 222, or 2222. Ask them to draw a model of their problem to solve it. Display all of the amazing word problems that students created. 

Here's how one 4th grader approached this task:

IDEA #21 (grade 3 standard 3.MD.C area and perimeter)

Give students 22 one-inch tile blocks. Guide them through this area/perimeter activity through questioning and discussion: 

• Can you arrange these blocks so they make a rectangle? (give plenty of time to explore - students may likely attempt arrays that are more square-like and discover that they cannot use all, so will need time to discover and rethink their ideas - try not to rush this important part of the learning) 
  NOTE: Students will be able to make an 11 by 2 or a 22 by 1 rectangle.

• Discuss the various configurations that students created. 
• Chart the various models as you discuss them. 
• Ask, "What is the area of the rectangle?" (add the information to the chart)
• Ask, "What is the perimeter of your rectangle?" (add to the chart)
• Challenge students to make composite figures using the 22 unit squares.
• Sketch and chart the information. 
• Ask what generalizations can be made about the area and perimeter relationship? Below are some sample ideas that your students may name:
• The area is always 22 not matter how the units are configured
• The more the shape looks like a square, the smaller the perimeter
• The area is measured in "square units"
• The perimeter is measured in "units" (not squared since we are measuring the one dimension of length)

...and finally... 
IDEA #22 is, of course, targeted to 2nd GRADE content (grade 2 standard 2.NBT.A.4)

Students in second grade and beyond are expected to be able to compare any 3-digit number based on place value. Show students the comparison statement 11=11 (eleven equals eleven). Tell them that since today is a very special TWOsDay, you have two 2s that you are going to squeeze in as digits with each of the numbers shown. Challenge them to not only tell which number is now greater, but to explain how the location of the 2 impacted each number. 

11 = 11 after inserting a 2 digit into each number, show students 211 ?  121. Notice that both 3-digit numbers have two ones and one two. But which one has the greater value? Give your students time to discuss their ideas with a partner then select and discuss some of their ideas with the whole class. Use inequality symbols to show the correct number sentence 

211 > 121 

Now move the 2s to different locations (112 ? 211). Discuss the new inequality. Perhaps change the 1s to 9s and repeat the process. 

Just for fun, I set this post to publish on 2/2/22 at 2:22 am

THANK YOU, Steve O. for encouraging me to get this resource out there - it was on my mind but may have fallen to wayside had you not given me that little push last week :) 

Accelerate vs. Remediate

I had a conversation the other day that is likely a common one, but why it’s common (or even an acceptable practice) is a mystery to me – I’ve accepted it as “normal” for so many years – recently, however, a very bright lightbulb lit up in my brain 💡, and I finally have enough light to see a better path....

I’m not sure that there was a specific “thing” that flipped the switch to light the path, but here’s an interaction that was the driving event behind this month’s post.

I saw the special education teacher in the hall and inquired about the progress of a student we had previously discussed. 

Me: Hi, Mr. Soenso. How did Jenny do trying the compensation strategy? Did it help her wrap her head around subtraction a bit better? 

Special Education Teacher: Yeah, it was a good start. Today I’m working with Jenny on rounding because she struggled with it during math class yesterday. She has always struggled with place value concepts, so I’m on my way to pull her for some remedial support now.

I'm willing to bet that conversations like this one take place in your school, too. They are so common, in fact, that you may be scratching your head trying to figure out what I'm trying to highlight by sharing this interaction. 

Often when we provide instructional support to students, we “put the car in reverse” and back up to the things the student was unable to do during regular instruction while the rest of the class continues to drive forward.

I was talking about this observation with a colleague, and she said, “This reminds me of a book I’m reading, Learning in the Fast Lane. I think you'd like it. The beginning part of the book aligns exactly with what you are saying.” So, I, of course, got a copy of the book, and, sure enough, the author put many of my thoughts right in the opening chapters of her book. 

My conversation with the special education teacher was not unique to the role of special educators or to students with IEPs; we do the exact same thing in our general education classes during small group instruction, too. We plan our small groups based on who was or was not successful on the lesson that we taught to the whole group – I know this is pretty typical because I planned the small groups in my own classroom using this exact same model for many years.

It looked something like this: I knew that some of my students did not have the foundational knowledge that would be needed for the next lesson, and it was unlikely that they would "get it" during whole group instruction, so, in my plans, I included small group instruction that would follow the whole-group instruction. I would group these students together with the plan of going over the material with them again while their classmates completed independent practice work or played partner games to reinforce the concepts. 

Now let’s consider an alternate (yes, better!) plan: I knew that some of my students did not have the foundational knowledge that would be needed for the next lesson, and it was unlikely that they would "get it" during whole group instruction, so, in my plans, I included small group instruction that would be taught the week BEFORE the whole-group lesson. During this small group instructional time, I could work with the students who I already knew needed more support learning the specific foundational skills required to fully participate in next week's whole-group lesson. And because they arrive in the whole-group lesson armed with new, relevant foundational knowledge, they participate more, they connect ideas more, they learn more. They are not trying to catch up to their classmates during whole group instruction; they are working alongside of them. 

This way of planning instruction is referred to as acceleration. It takes a pro-active approach to small group instruction and is designed with the expectation that the whole group will be able to engage in the whole-group lesson during core instruction. Acceleration enhances many skills: (1) Students gain increased ability with the skill; (2) they have a broader understanding of one or more strategies; and, most importantly, (3) student confidence increases during the on-grade level whole group lesson because the student had an opportunity to PREview the skills and strengthen foundational skills before that whole-group lesson occurred -- and student confidence probably has the greatest effect of all when it comes to learning and achievement. 

Think about the structure and purpose of the acceleration lane on the highway.... The acceleration lane is designed to help drivers "get up to speed" so they can merge smoothly into the flow of traffic. Similar to the acceleration lane on the highway, the purpose behind using acceleration practices/strategies in the classroom is to help our students "get up to speed" on the specific foundational skills required to engage in the upcoming lesson WITH their peers (in the flow of traffic), not after their peers have zoomed past them on the highway of learning.

Let’s talk ACCELERATION vs. REMEDIATION

The term “acceleration” is one that has been around for a while. If you’re like me, you haven’t used it the way it is currently being used in educational circles. So for the purpose of this post, let’s establish a common definition: 

For me, acceleration previously brought images of students doing work that is beyond the scope or depth of what is considered typical for their grade level peers. My school district’s Accelerated Math 8 class, for example, allows students to work on their high school Algebra content while still in middle school.  We will, however, be using the term acceleration differently today – and we’ll mention the term remediation, too. Here is how remediation and acceleration are described in Suzy Pepper Rollins' book Learning in the Fast Lane:

The primary focus of remediation is mastering concepts of the past. Acceleration, on the other hand, strategically prepares students for success in the present—this week, on this content. Rather than concentrating on a litany of items that students have failed to master, acceleration readies students for new learning. Past concepts and skills are addressed, but always in the purposeful context of future learning (p.6).

Remediation is based on the misconception that for students to learn new information, they must go back and master everything they missed (p.5). By backing students up to reteach “everything”, we are not closing the gap at all, in fact, we are widening it! As the gap gets wider and wider and students fall farther behind, motivation decreases and we now have a student who not only struggles in math, but actually hates it.

Let’s be PRO-active rather than RE-active

If we anticipate which students will need support with the foundational skills that are necessary to actively engage in the whole-group lesson, we can (and should!) use our small group time helping these students gain those “just in time” skills in the days prior to the whole-group lesson. Rather than allowing these students to sit through an entire lesson, gain nothing, and then have to repeat (not only that lesson but also provide instruction to address foundational gaps), why don't we spend our time more effectively by anticipating the needs and meeting those needs before the grade-level lesson.

So HOW do we get started?

Step 1: Shift our mindset about small group instruction. I believe small group instruction is important, but I don’t think that every student needs it every day for the exact same amount of time. For some of my students, small group time is the optimal time to work on just-in-time foundational skills that will enable them to participate (with their peers!) in next week’s whole group lesson.

Step 2: Determine which skills are foundational to next week’s lesson. You may be wondering how to do this? In math, I rely on Achieve the Core’s Coherence Map tool. First I select the standard that will be addressed next week during the whole-group lesson. Then I simply click on the linked standards that are foundational to the selected standard (look at the standards to the left when viewing the Coherence Map). And that brings us to Step Number 3.  

Step 3: Assess which students will need additional support with foundational skills necessary to access the upcoming lesson. This can be a formal or informal process. I prefer quick formative assessments and the wealth of teacher data I collect just from being observant and listening to my students share their mathematical ideas.

Step 4: Plan small group instruction that will occur PRIOR to whole-group instruction. This time should be purposeful in its attempt to level the playing field for your students so everyone can engage with the content and join the discussion during the upcoming whole-group lesson.

Step 5: Engage the targeted students in activities that create BRIDGES that will CONNECT the work done in small group to the grade level standard that will be taught in the coming days during whole group. And remember, the targeted group of students should not be a static list – during Step 3, consider which students need support with these specific skills. 

IMPORTANT NOTE: And if you have a co-teacher (special education teacher, Title I teacher, instructional assistant, etc.) who delivers some or all of your small group instruction to any of your students, be sure to communicate daily with that teacher so they, too, can plan small group instruction that is that is both pro-active and aligned to the upcoming core lesson.

Drivers, start your engines and let's get in the "FAST LANE"!